Notes
divided square solution

Solution to the Divided Square Puzzle

Solution by Area of a Triangle and Vertically Opposite Angles

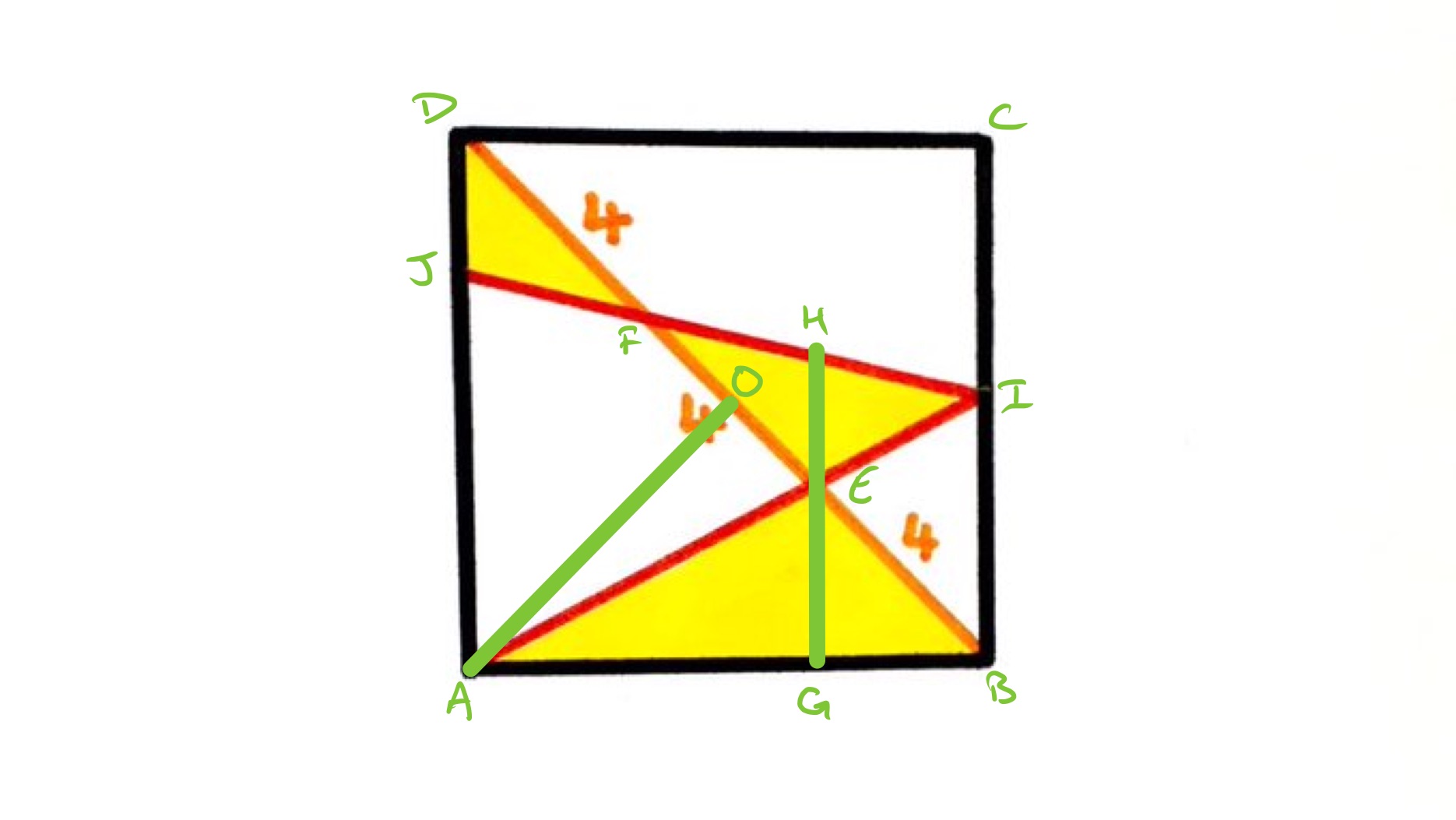
With the points labelled as above, consider first triangle $A B E$. The length of $E B$ is given as $4$. The height of $A E$ above $E B$ is the length of $A O$, which is half a diameter and so is $6$. Therefore the area of triangle $A B E$ is $12$.

Since $E$ is $\frac{2}{3}$rds of the way along $D B$, $E$ is also $\frac{2}{3}$rds of the way along $A I$, so $E I$ is half the length of $A E$. As angles $O \hat{E} I$ and $A \hat{E} B$ are equal, since they are vertically opposite, the height of $I$ above $E F$ is then half the height of $A$ above $B E$, so the area of $E I F$ is half that of $A B E$, so is $6$.

The line $H G$ is vertical through $E$, and so as $E$ is the midpoint of $F B$, $G$ is the midpoint of $F I$. This means that triangle $E H F$ has half the area of triangle $E I F$, so has area $3$. Then angles $D \hat{F} J$ and $E \hat{F} H$ are equal as they are vertically opposite and $D J$ is parallel to $E H$ and $D F$ and $F E$ are the same length, so triangles $D F J$ and $E F H$ are congruent. Hence triangle $D F J$ has area $3$.

The shaded region therefore has area $12 + 6 + 3 = 21$.