Notes
circle in a quarter circle solution

Circle in a Quarter Circle

Solution by Pythagoras' Theorem and Area of a Circle

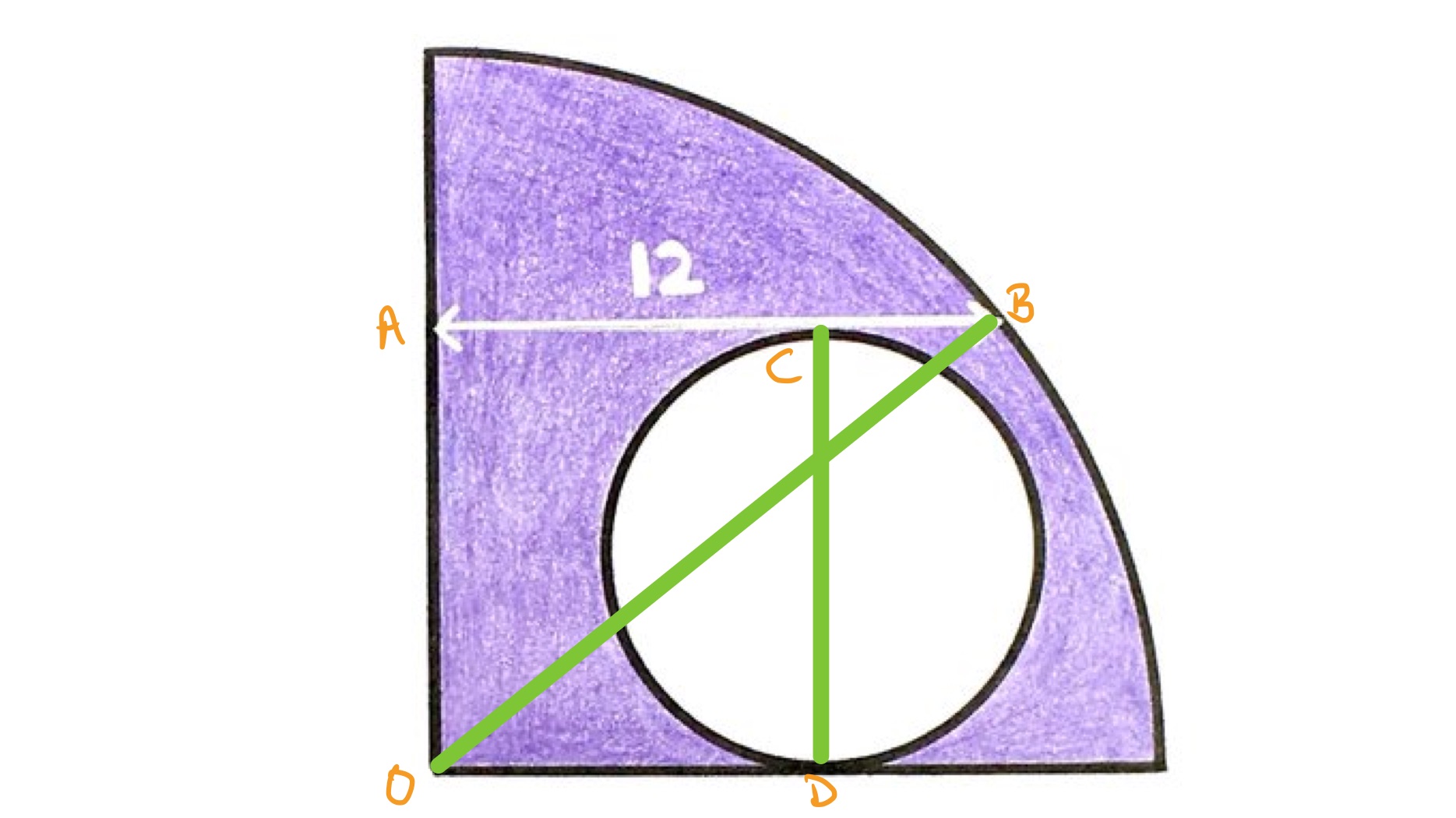
Let $R$ be the radius of the quarter circle, which is the length of $O B$, and let $r$ be the radius of the smaller circle, so the length of $C D$ is $2 r$. The lengths of $C D$ and $A O$ are the same, so applying Pythagoras' theorem to right-angled triangle $O A B$ shows that:

The area of the quarter circle is $\frac{1}{4} \pi R^2$ and the area of the inner circle is $\pi r^2$, so the area of the shaded region is:

So the area of the shaded region is $36 \pi$.