Notes
chord and annulus solution
Chord and Annulus
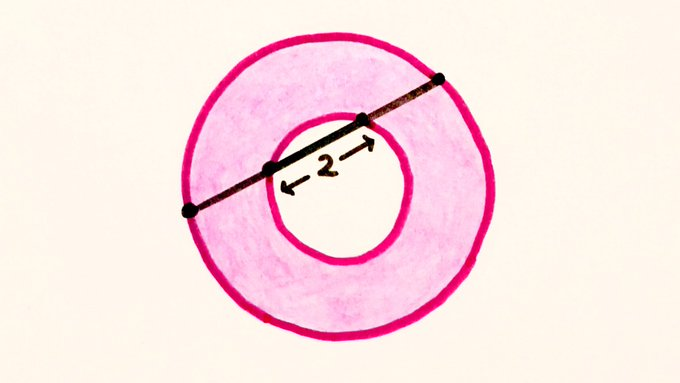
The four dots are equally spaced. What’s the shaded area?
Solution by Pythagoras' Theorem
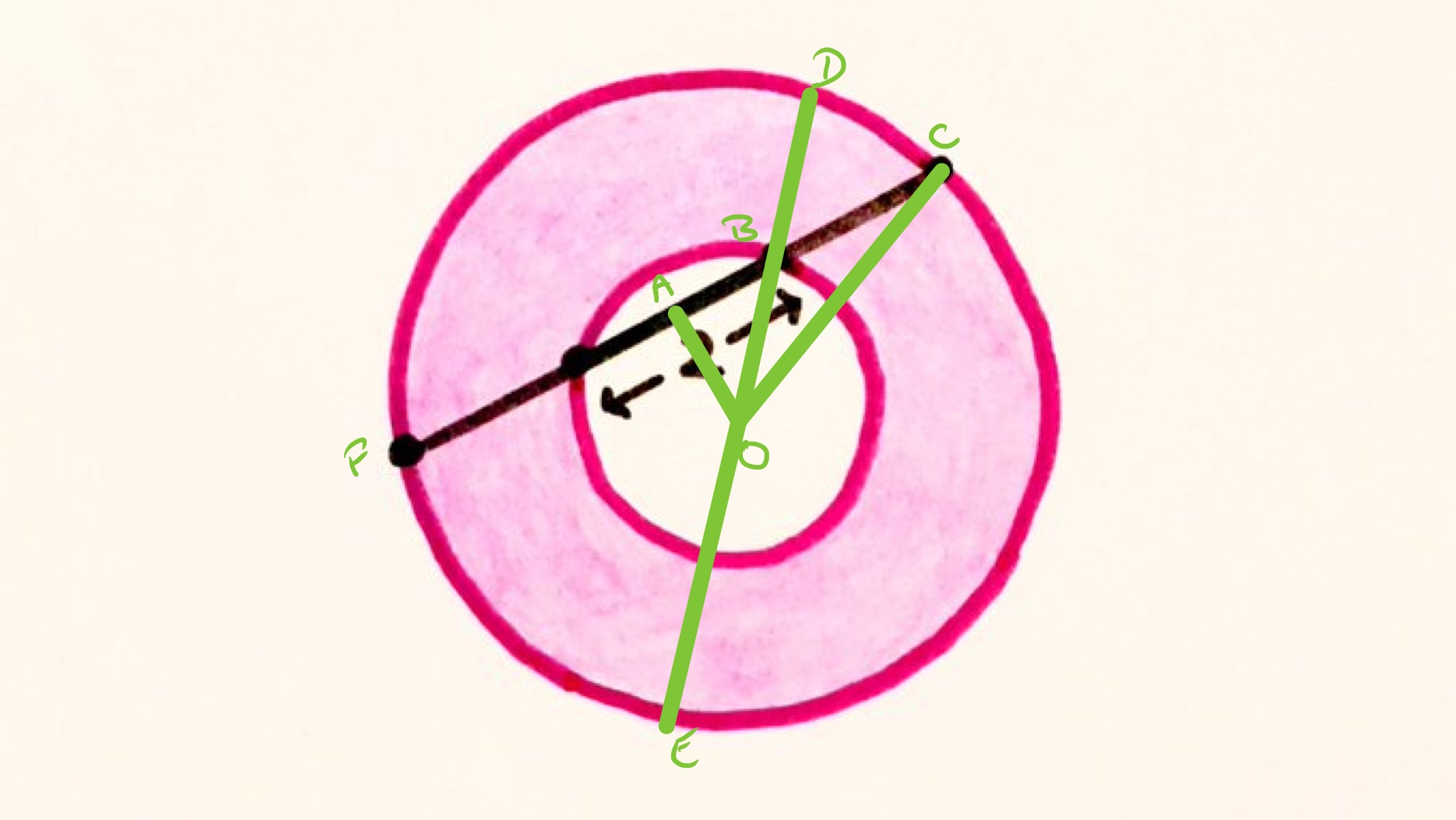
With the points labelled as above, let be the radius of the inner circle and of the outer. The shaded region has area .
Point is the midpoint of the inner chord, so angle is a right-angle. The length of is . Let be the length of . Applying Pythagoras' theorem shows that .
Since the dots are equally spaced, has length . Applying Pythagoras' theorem to triangle shows that . Therefore and so the shaded region has area .
Solution by the Intersecting Chords Theorem
With the points as in the the above diagram, and and also as above, the intersecting chords theorem applies to the chords and of the outer circle. The relevant lengths are those of , which is , of , which is , of , which is , and of , which is . Putting these together shows that . As above, this gives for the area of the shaded region.