Notes
angle in a circle and semi-circle solution
Solution to the Angle in a Circle and Semi-Circle Puzzle
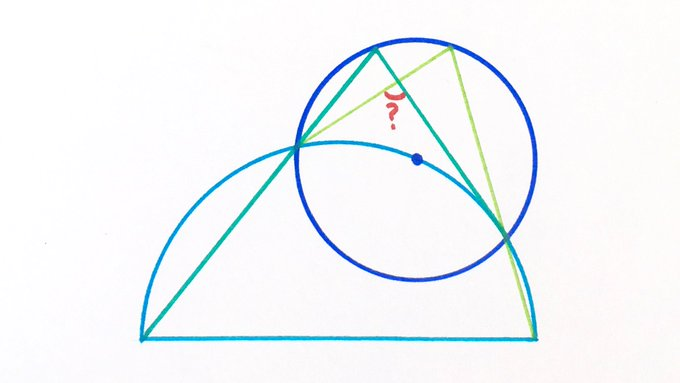
What’s the angle?
Solution by Angles in a Cyclic Quadrilateral, Angle at the Centre is Twice the Angle at the Circumference, Angles in a Triangle, Angles at a Point on a Straight Line, and Vertically Opposite Angles
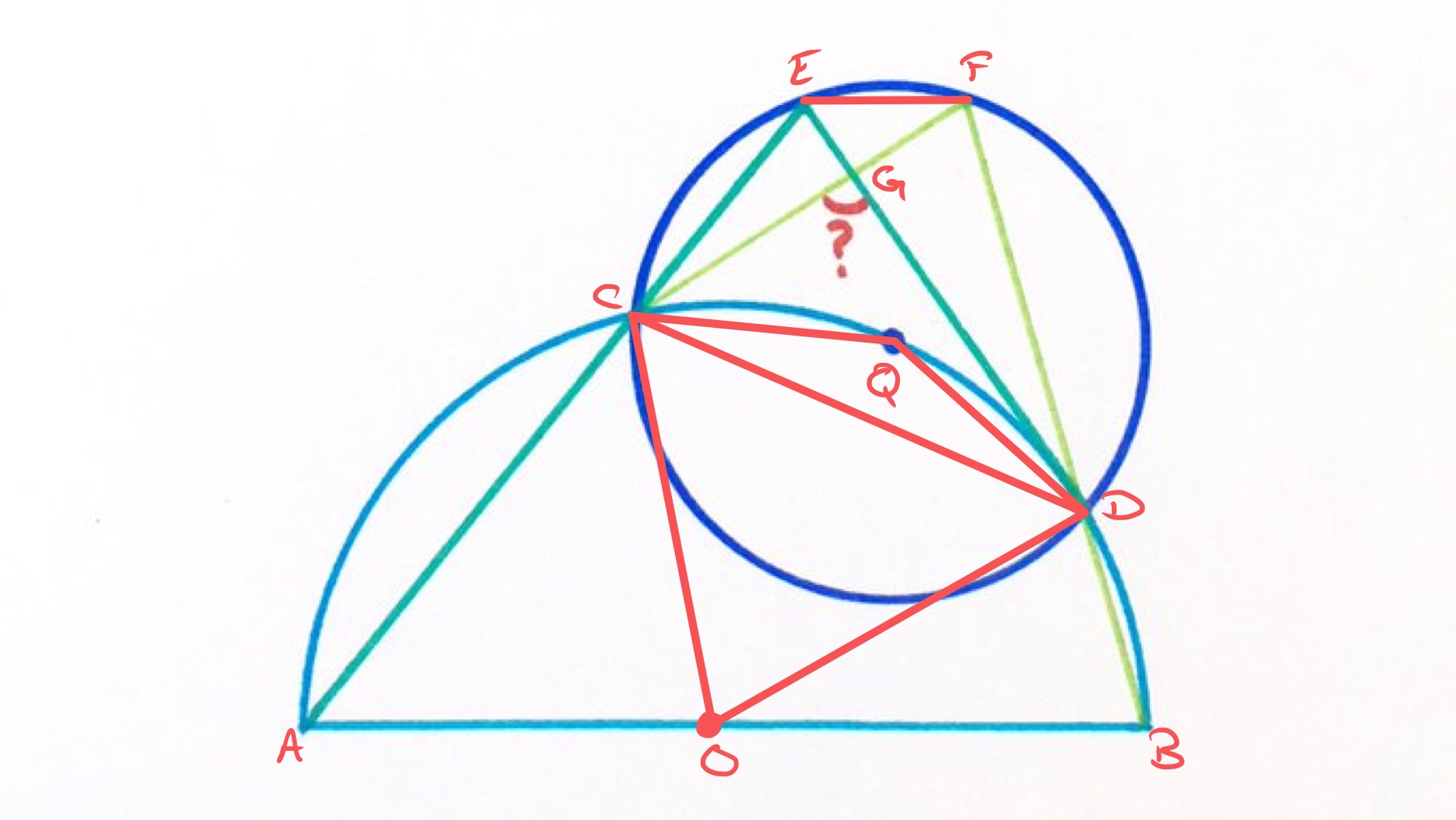
In the above diagram, the point labelled is the centre of the semi-circle. Let angle be and let be .
Since opposite angles in a cyclic quadrilateral add up to , angles and add up to , so angle is . Then as angles at a point on a straight line also add up to , angle and add up to , so is equal to angle . Using opposite angles in a cyclic quadrilateral again, angles and add up to meaning that angle is . A similar argument shows that angle is . The sum of angles and is therefore .
As triangle is isosceles and the angles in a triangle add up to , angle is . Using a similar argument gives angle as . Therefore the reflex angle is . Since the angle at the centre is twice the angle at the circumference, the reflex angle is . Applying this again shows that this is twice each of the angles and . So the sum of the angles and is angle .
The sum of angles and is then the difference between these two sums, so is . Since the angles in a triangle add up to , angle is . Therefore as vertically opposite angles are equal, angle is .