Notes
angle formed by two squares ii solution
Solution to the Angle Formed by Two Squares II Puzzle
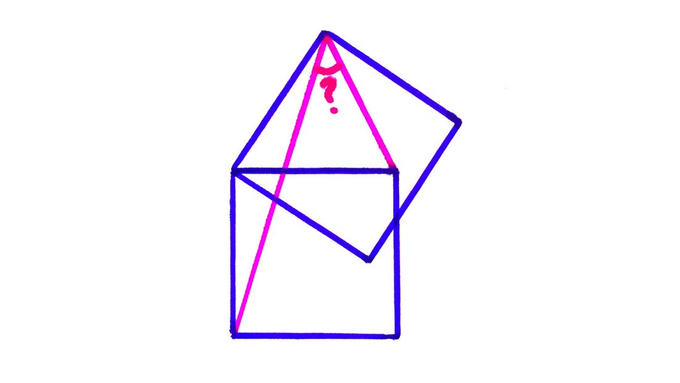
These squares are the same size. What’s the angle?
Solution by Isosceles Triangles
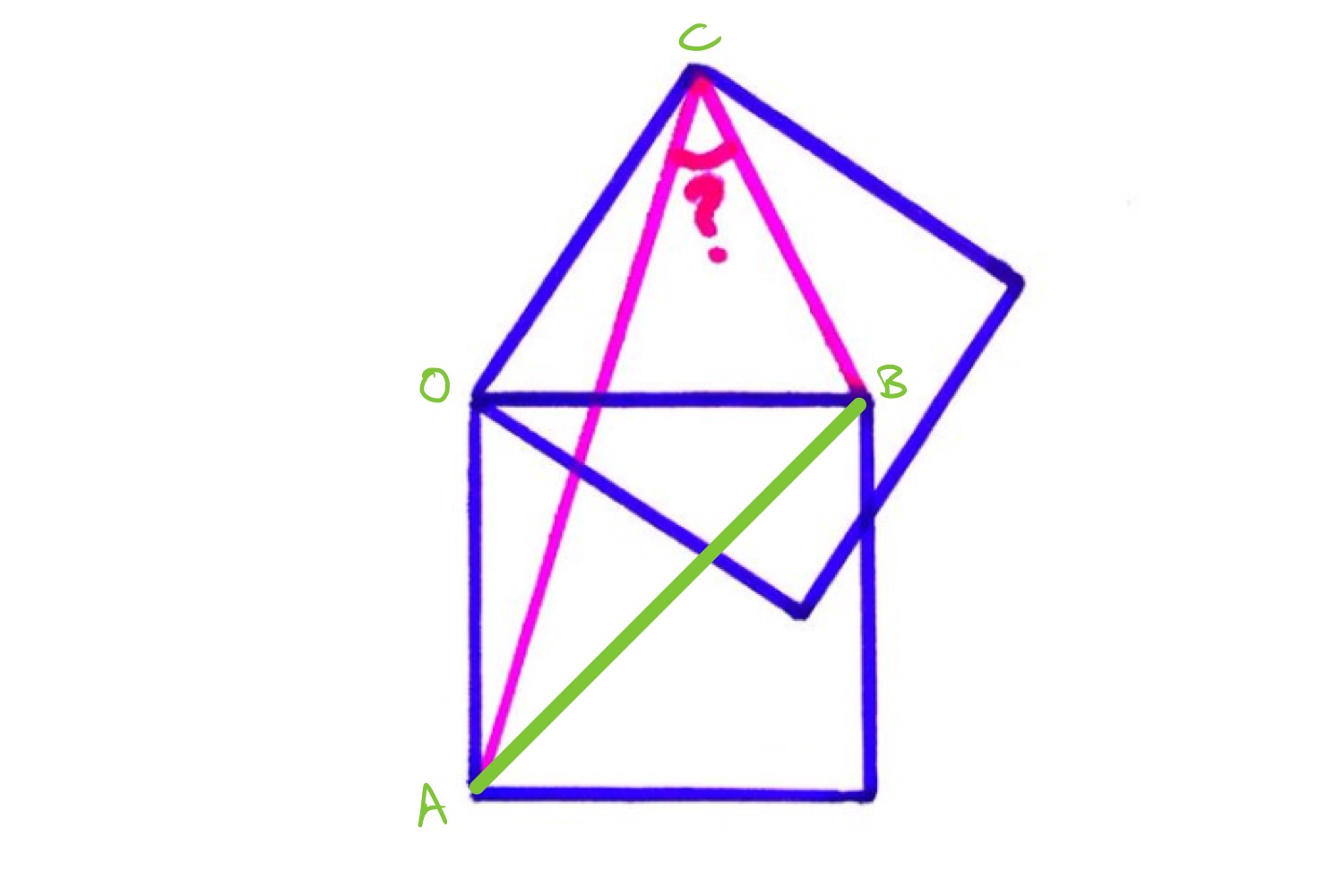
As the squares are the same size, triangle is isosceles with and of the same length. Also triangle is isosceles, with and the same length. Let be angle and let be angle . The requested angle is .
As is isosceles, and the angles in a triangle add up to , angle is . Similarly, angle is . Therefore, angle is
It is also the corner of a square, so is . Hence .
Solution by Angle at the Circumference is Half the Angle at the Centre
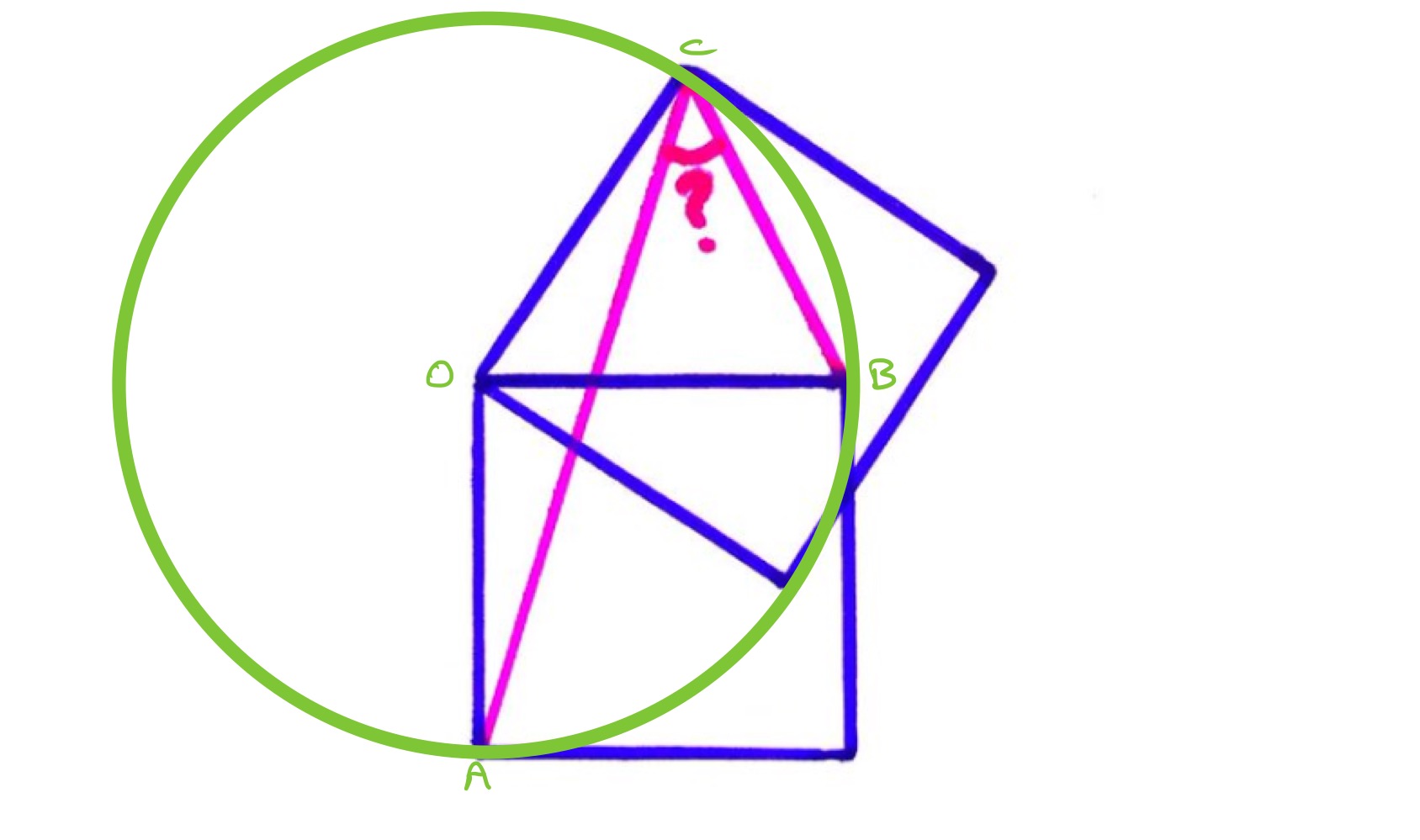
As the squares are the same size, the circle centred at that passes through also passes through and . Then the requested angle is half of angle , which is , since the angle at the circumference is half the angle at the centre.
Solution by Invariance Principle
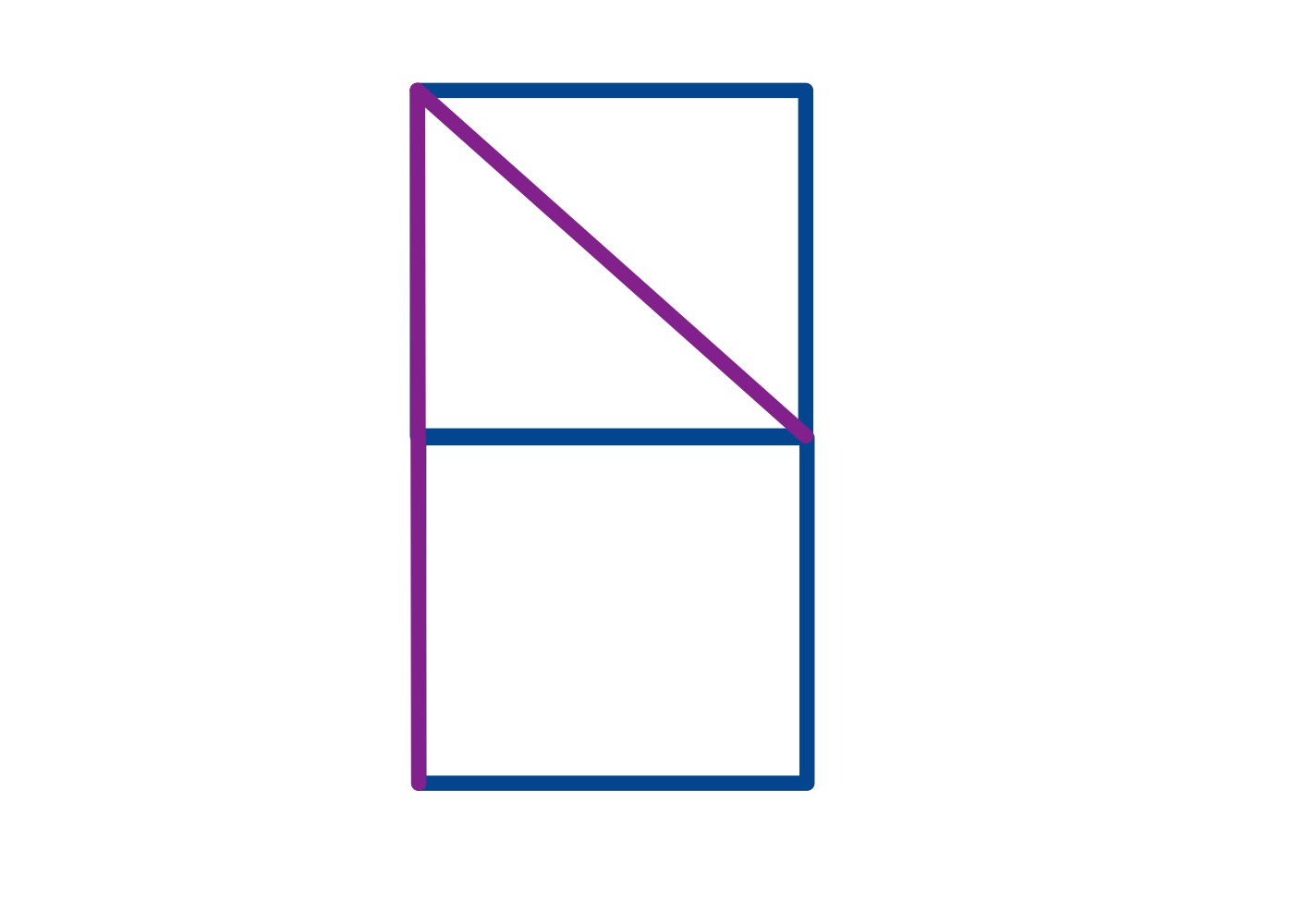
By drawing the squares so that they are adjacent without overlapping, the requested angle can be seen to be .