Notes
a square, a circle, and a rectangle solution
A Square, A Circle, and a Rectangle
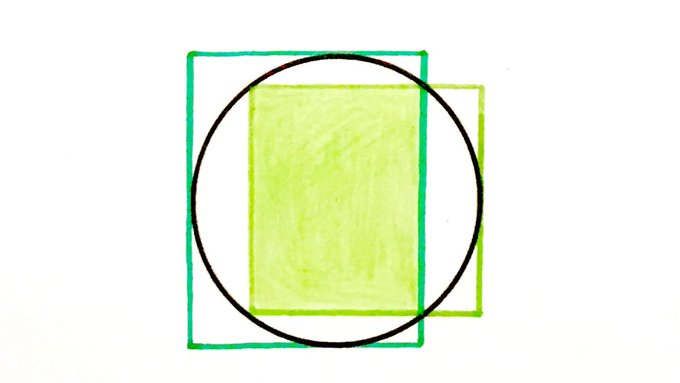
A square, a circle and a rectangle. What fraction of the total area is shaded?
Solution by Pythagoras' Theorem and Lengths in Circles
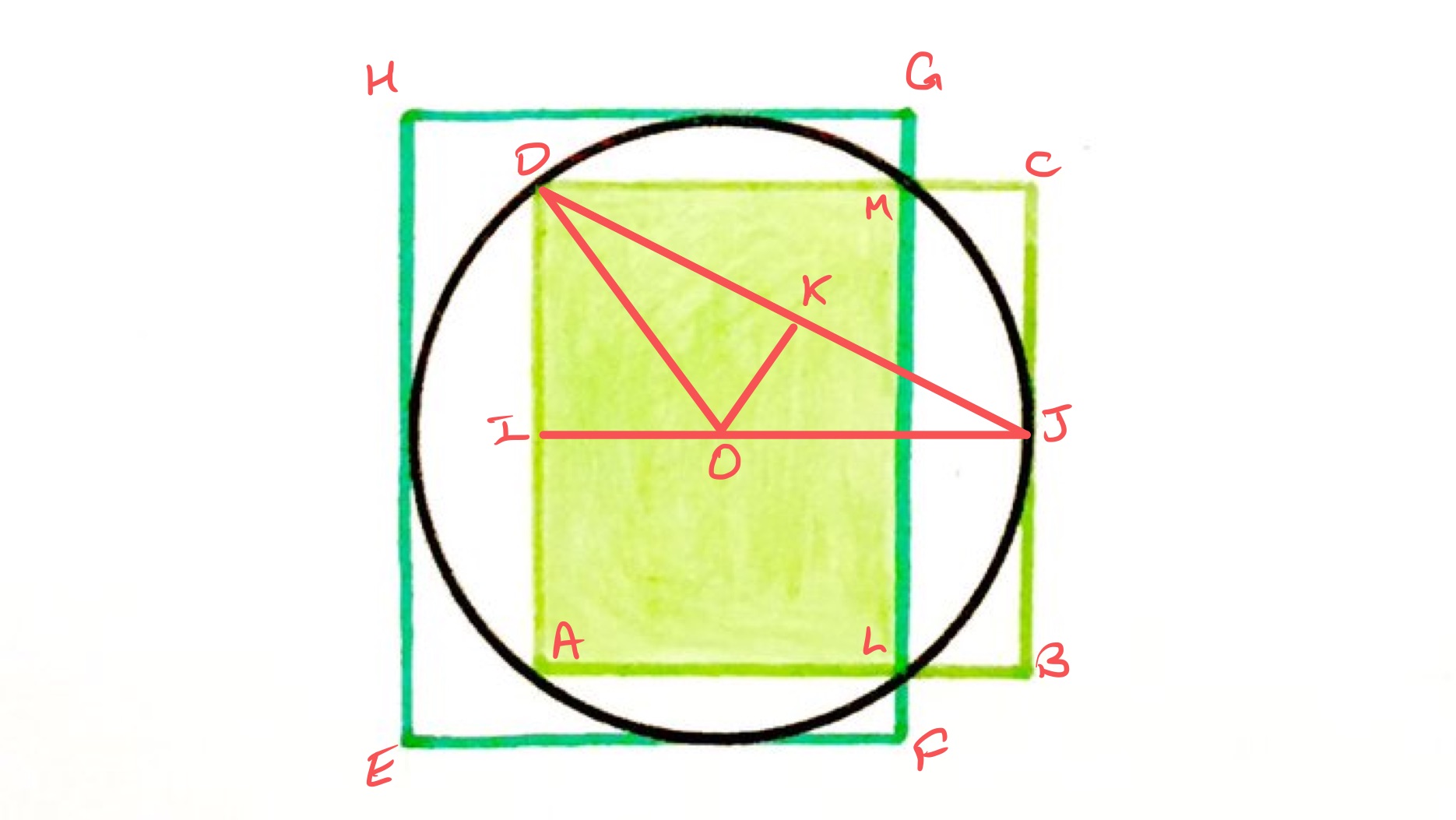
In the above diagram, the point labelled is the centre of the circle and the point labelled is the midpoint of the line segment .
Let be half the length of the side of the square, so that has length . Then triangle is a right-angled triangle with short sides of lengths and . Using Pythagoras' theorem, its hypotenuse is then .
Since and are both radii of the circle they are the same length. Therefore triangle is isosceles and so the line segment splits it into two equal right-angled triangles. As triangle shares an angle with triangle at , they are similar. The length of is so the length scale factor is . The length of is therefore .
Since has length , must therefore have length which means that has length . So the shaded area is a rectangle of dimensions and so has area . The outer rectangle has width and height so has area . Finally, the square has area .
The total area of the shape is therefore and so half of the full shape is shaded.