Created on August 13, 2025 11:30:29 by Andrew Stacey (81.109.65.126).
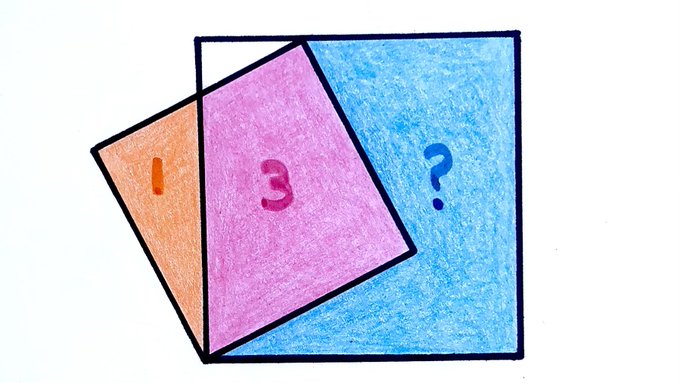
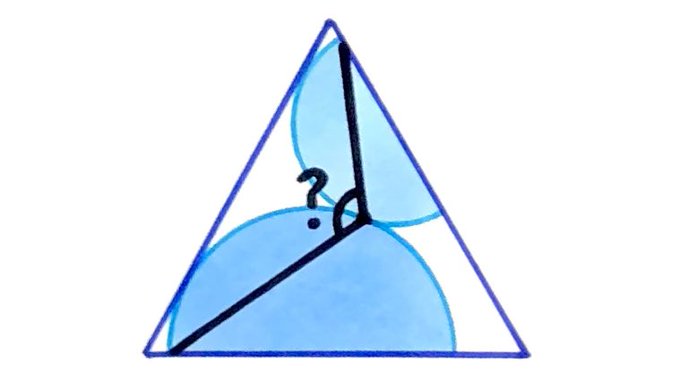
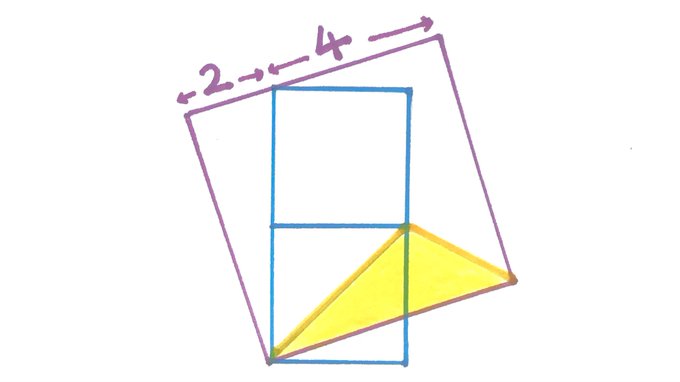
Two squares. What’s the missing area?
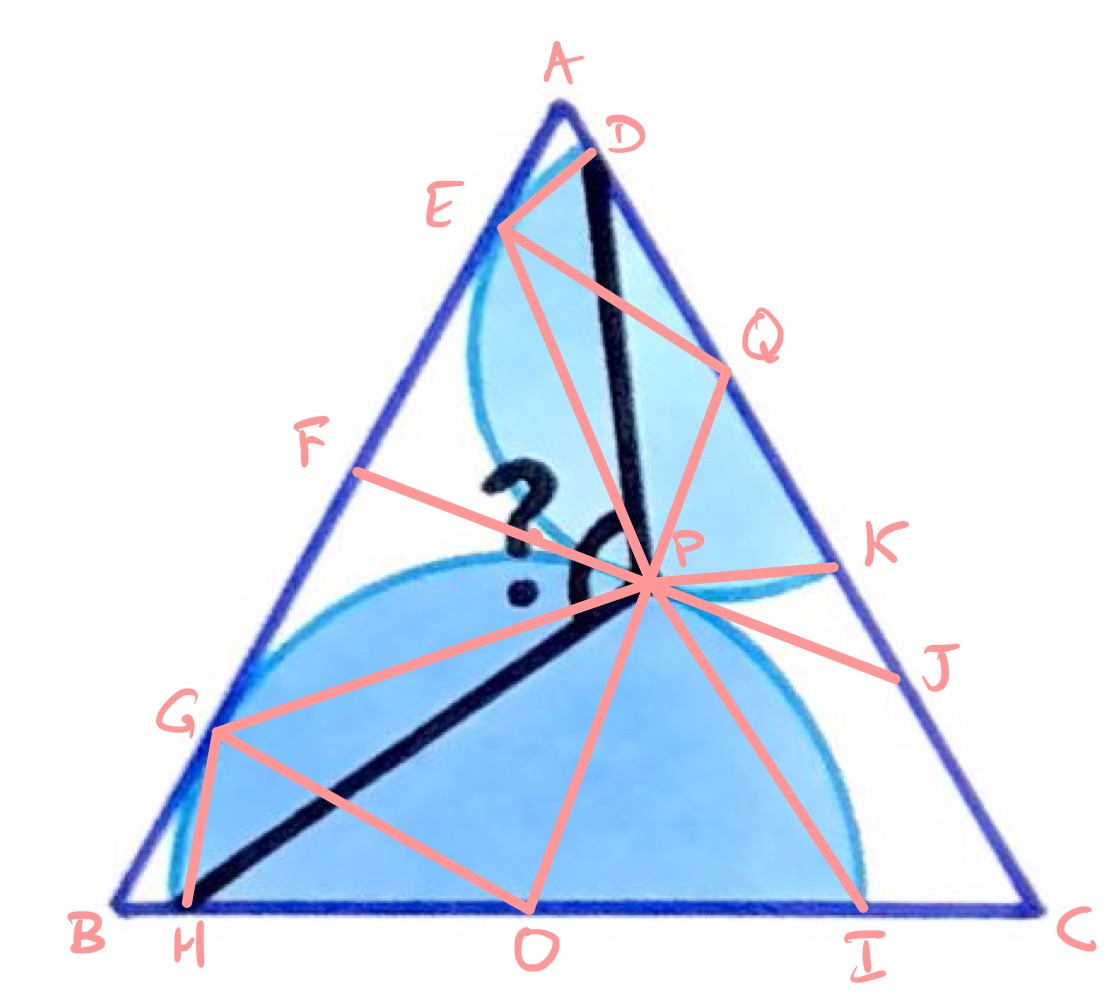
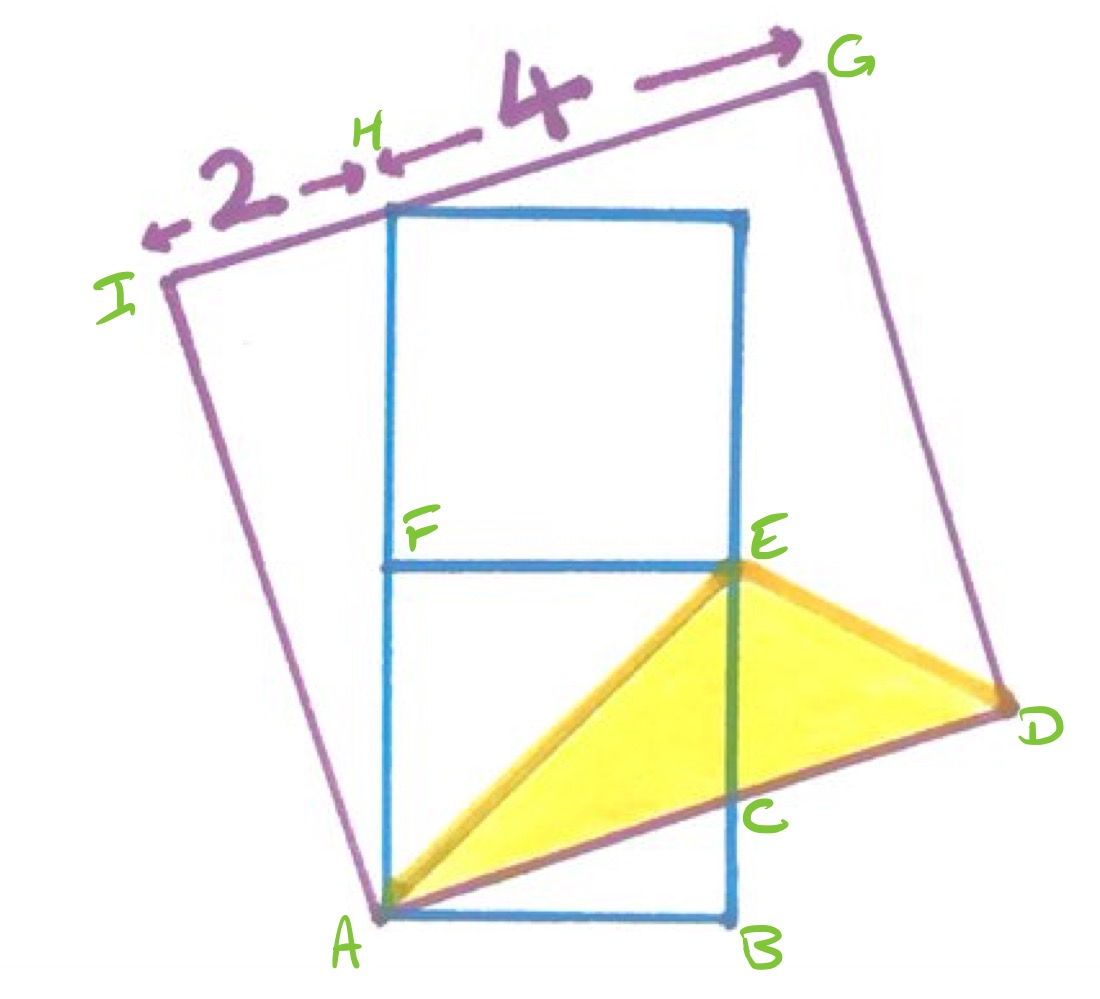
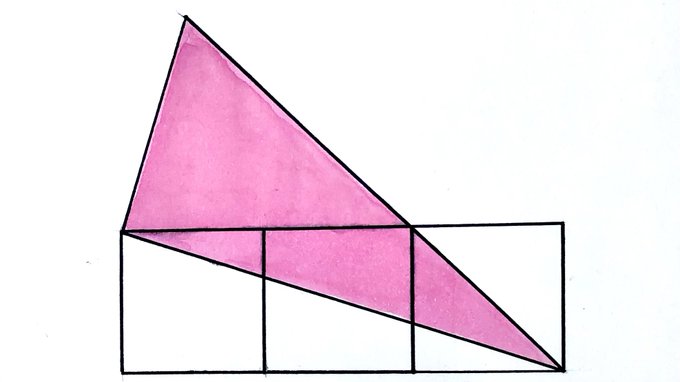
In the diagram above, the additional points are as follows:
- is so that is perpendicular to ,
- is so that is parallel to ,
- and are so that passed through and is perpendicular to ,
- is so that is perpendicular to ,
- is so that is the continuation of ,
- is so that is perpendicular to .
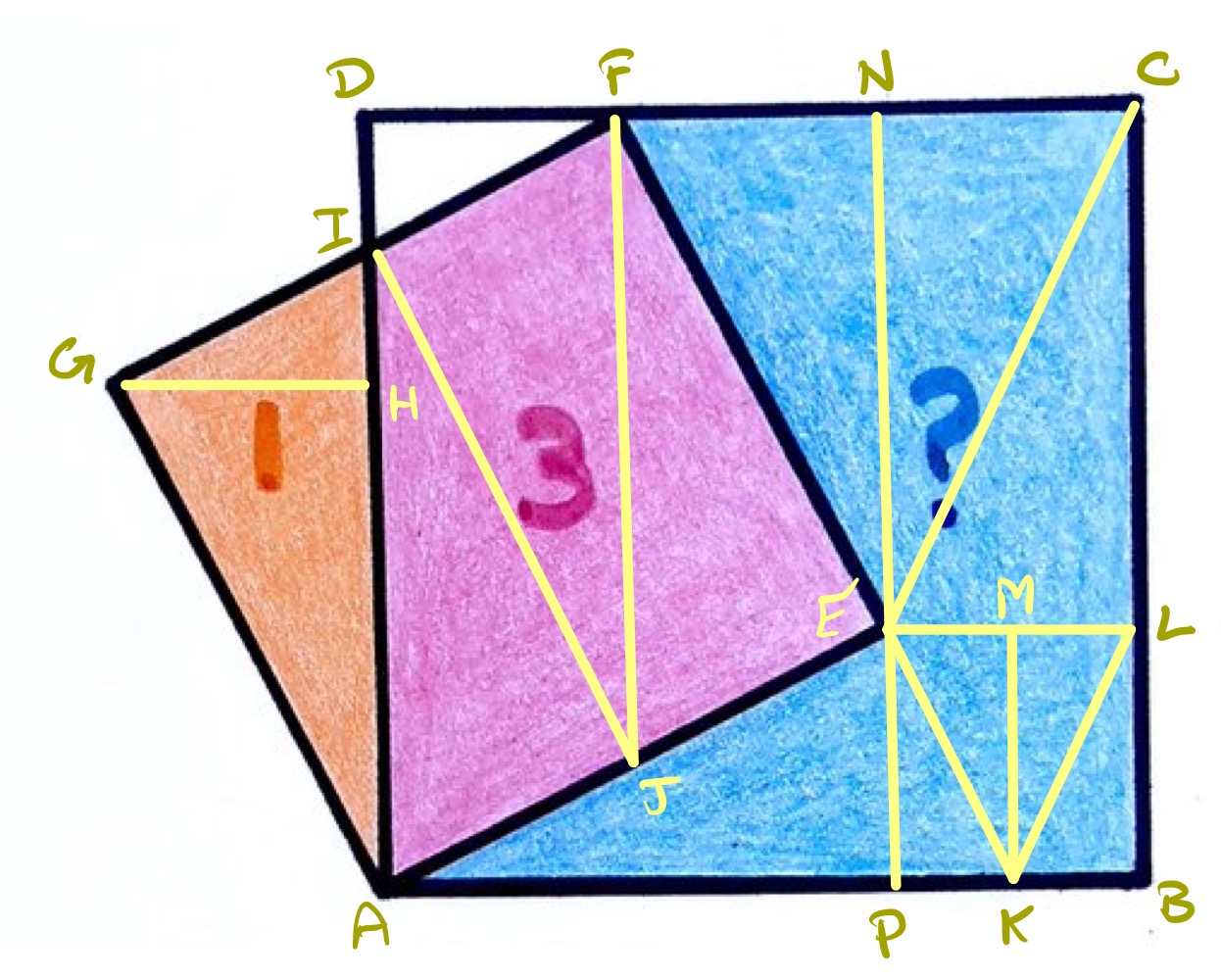
Since the tilted square, , has total area , its side length is . Then is a right-angled triangle with area and has length , so must have length . This means that is the midpoint of , and then that is also the midpoint of .
Since is parallel to , this means that is also the midpoint of , and then that is parallel to . So the decomposition shown of the purple region is into three congruent triangles, each of area .
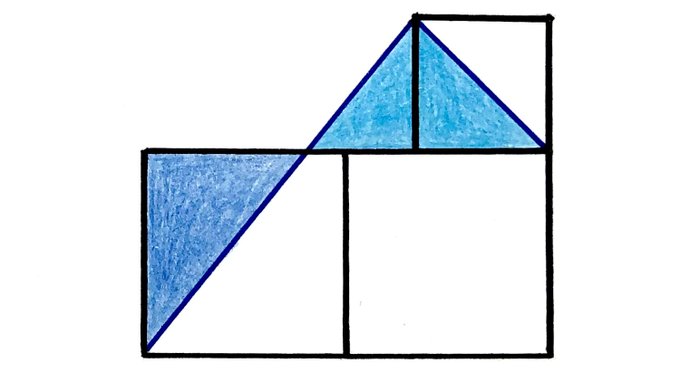
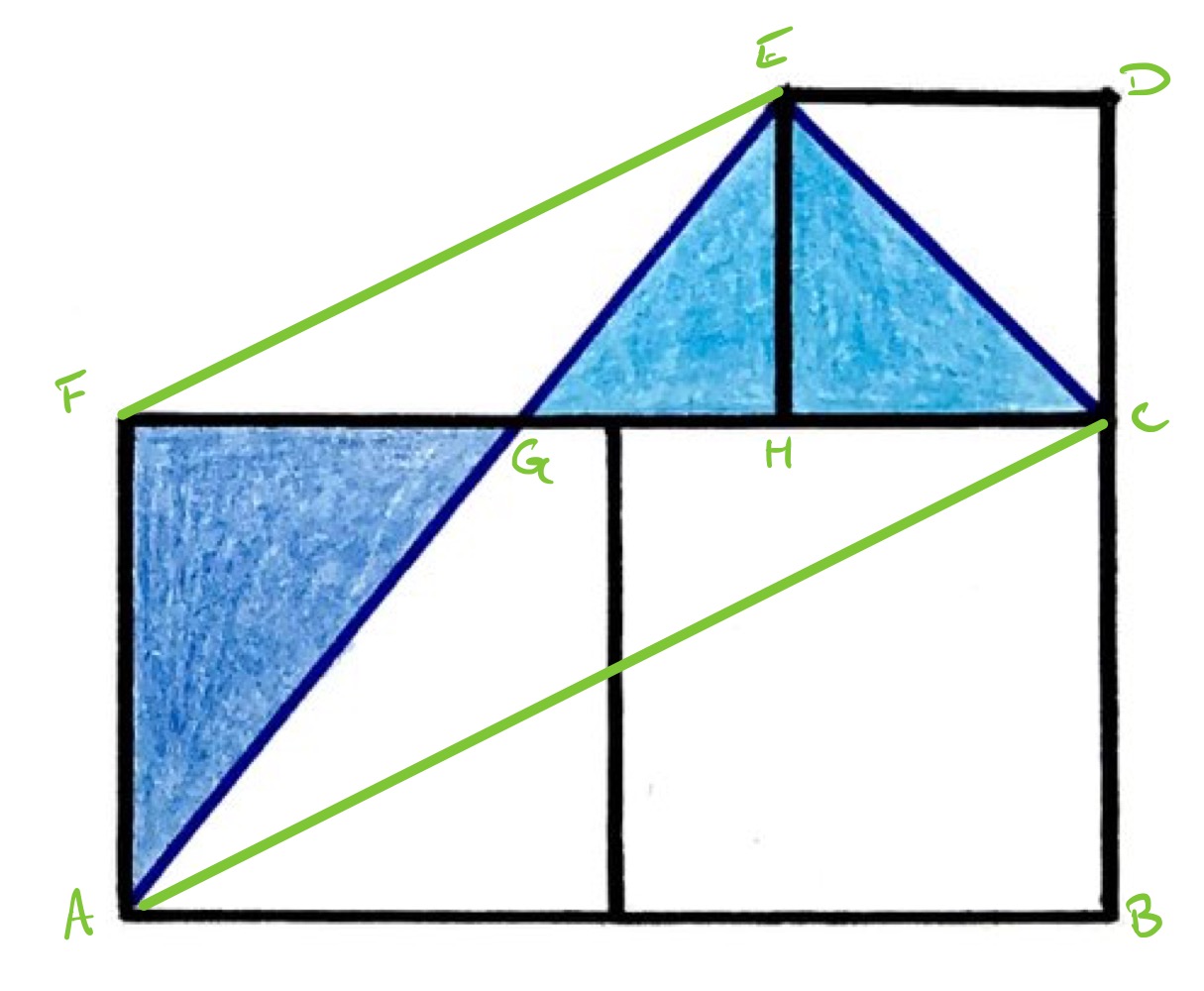
Since is the continuation of , triangle is right-angled. Then as has the same length as , and angles and are the same, triangles and are congruent. Therefore, line segments and have the same length, so since and are sides of the same square, line segments and have the same length. Then line segment has the same length as , so triangle is congruent to triangle .
The congruency between and takes to , so triangle is also congruent to triangle , meaning that line segment also has the same length as , and so is the midpoint of . Since the lengths of and are in the ratio , this also holds for and , meaning that and have the same length, so then line segment has the same length as .
Triangle is right-angled, with parallel to and parallel to and of the same length. Therefore, triangle is congruent to triangle . Triangle is then also right-angled, shares with triangle , and line segment is the same length as line segment , which is the same length as . Therefore, is also congruent to . Since is a rectangle, triangle is also congruent to triangle .
In summary, triangles , , and are all congruent to triangle ; triangles , , are all congruent to triangle ; and triangle is congruent to triangle . The three smallest pair with the three middle to create regions of area ; then since this is also the area of triangle this gives a total area of .